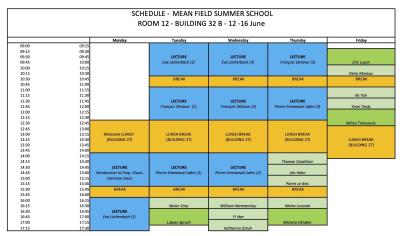
Lectures :

François Delarue (Univ. Nice Côte d'Azur) Mean field games and control

Pierre-Emmanuel Jabin (Pennsylvania State Univ.) Some new developments on the mean-field limits of non-exchangeable systems

Eva Löcherbach (Univ.Paris 1) Mean-field limits for systems of interacting and spiking neurons

Talks :

Thomas Cavallazzi (Univ. Rennes) Quantitative weak propagation of chaos for McKean-Vlasov SDEs driven by $\alpha$-stable processes

Antoine Diez (Kyoto Univ.) Introduction to propagation of chaos and mean-field models

Xavier Erny (École Polytechnique) Annealed limit and quenched control for a diffusive disordered mean-field model with random jumps

Leo Hahn (Univ. Clermont Auvergne) Invariant measure and mixing behavior of a pair of run-and-tumble processes with hard-core interactions

Yi Han (Univ. Cambridge) Stochastic PDEs on Hilbert space with irregular noise coefficients

William Hammersley (Univ. Nice Côte d'Azur) Regularising gradient descents on the space of probability measures with the rearranged stochastic heat equation

Pierre Le Bris (Sorbonne Univ.) An observation concerning the effect of the Random Batch Method on phase transition

Marta Leocata (Scuola Normale Superiore, Pisa) Some variations on the mean-field limit: different types of interactions and the first-order approximation

Eric Luçon (Univ. Paris Cité) How large is the mean-field framework? LLN and CLT for empirical measures of diffusions on (random) graphs

Rémi Moreau (Univ. Rennes) Constrained in law BSDE and associated particle system

Michela Ottobre (Heriot Watt Univ.) McKean-Vlasov S(P)Des with additive noise

Katharina Schuh (TU Wien) Global contractivity for Langevin dynamics with distribution-dependent forces and uniform in time propagation of chaos

Lukasz Szpruch (Univ. Edinburgh) Fisher-Rao Gradient Descent for Stochastic Control Problems

Yoan Tardy (Sorbonne Univ.) Convergence of the empirical measure for the Keller-Segel model in both subcritical and critical cases

Milica Tomasevic (CNRS - École Polytechnique) Particle approximation of the doubly parabolic Keller-Segel equation in the plane

Ke Yan (Univ. Rennes) Extended mean-field control problem with partial observation